GreenLab Course
Development
Stochastic axis development modelling
Rhythm ratio
-
The rhythm ratio wφ of a given axis (of physiological age φ) is defined as
the ratio of potential new phytomer occurrence to the potential occurrence on an axis of physiological age 1.
The rhythm ratio wof a given axis characterizes the relative alternating periodic rests and activity.
The rhythm ratio is modelled as a periodic rest.
In other words it defines a pattern of periodic sequences (1 and 0), in which pattern 1 stands for potential phytomer appearance (in fact a slot of a Bernoulli trial), and 0 for a rest (a phytomer will never appear on its corresponding growth cycle).
As an example, an axis with a rhythm ratio of 0.5 will develop twice as slowly as the main axis (assumed to be the reference with w = 1), and the rhythm ratio will be modelled by the following sequence 1010.. 10...
This model is in fact the definition of a discrete linear stair function:
yi = w . xi
- where xi = 1, 2, 3, 4, .... stand for the consecutive growth cycles,
and yi stands for the number of Bernoulli trials.
The slope increment is known to be expressed as a period of successes (1) and failures (0) where w is defined by the number of successes divided by the number of successes and failures.
Depending on the w value this period can be more or less long, leading to approximations if a short number of cycles is considered.
For instance, a rhythm ratio of 0.6 defines a 5 step period: 11010
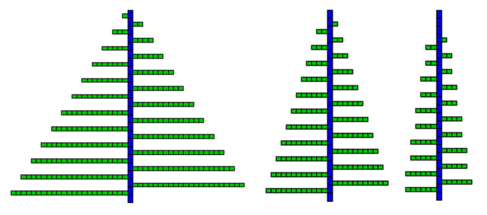
Various rhythm ratios (Images P. de Reffye, CIRAD)
- Left: both physiological ages 1 and 2 develop phytomers at the same rhythm;
the rhythm ratio w2 of the physiological age 2 axis is 1.
Middle: w2 is set to 0.5
Right: w2 is set to 0.25
